Hans Walser, [20130411a]

Motivation: M. N. D., N.

Golden Angle

1 Two angles

First we inscribe a pentagon into a square (Fig. 1a) and insert the blue angle .

Then we draw a diagonal in the Golden Rectangle ([Walser 2001, p. 34], [Walser 2013, p. 53]) and mark the blue angle (Fig. 1b).

Fig. 1: Two angles

The conjecture is .

2 Proof

2.1 The pentagon in the square

For the pentagon in the square we use the notation of Figure 2. The origin of the coordinate system is the centre of the Pentagon, which is not the centre of the square.

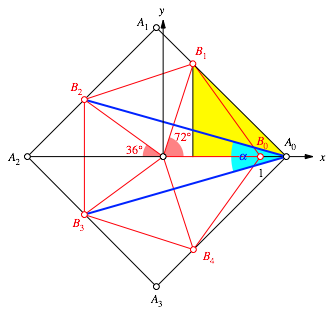
Fig. 2: Coordinate system

We have , , and .

Therefore:

For the Golden Section we use the usual notation:

(1)

It is easy to prove:

(2)

We can reduce every square of to a linear expression in .

Using ([Walser 2001, p. 54], [Walser 2013, p. 77])

(3)

we get:

(4)

2.2 In the Golden Rectangle

Figure 3 depicts the situation in the Golden Rectangle.

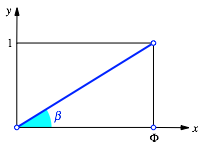
Fig. 3: The Golden Rectangle

We get:

(5)

Because of

(6)

and (2) and (5) we have:

(7)

2.3 Comparison

We compare (4) and (7):

(8)

This is equivalent to:

(9)

On the right hand side we have, using (2):

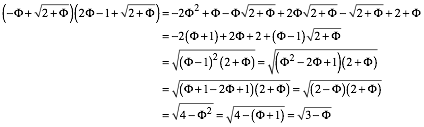
This is equal to the left hand side of (9). Hence .

Numeric result:

References

[Walser 2001] Walser, Hans: The Golden Section. Translated by Peter Hilton and Jean Pedersen. The Mathematical Association of America 2001. ISBN 0-88385-534-8

[Walser 2013] Walser, Hans: Der Goldene Schnitt. 6., bearbeitete und erweiterte Auflage. Edition am Gutenbergplatz, Leipzig 2013. ISBN 978-3-937219-85-1