1 About

The Knauth Figure (fig. 1) in the context of the theorem of Pythagoras.

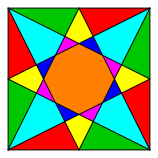
Fig. 1: The Knauth Figure

We will find differences between global symmetry and local symmetry.

2 The theorem of Pythagoras

We try to find a dissection proof of the theorem of Pythagoras in the special case of figure 2.

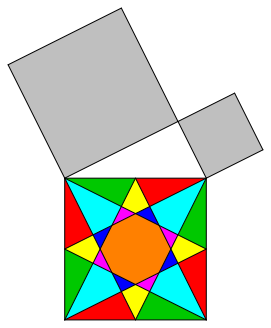
Fig. 2: Theorem of Pythagoras

3 Knauth tessellation

We extend the Knauth figure to a tessellation (fig. 3), where we cut out the three squares of the theorem of Pythagoras.

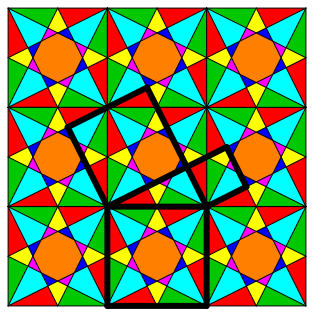
Fig. 3: Tessellation

This gives a dissection of the figure of Pythagoras (fig. 4). We still see the pattern of the global tessellation in the figure.

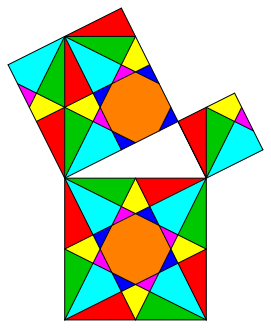
Fig. 4: Dissection

In this dissection we have a global symmetry due to the tessellation of figure 3. Every part fits in to the global pattern of figure 3.

But the dissections of the two small squares have no symmetry at all.

And there is another problem. We cannot move every individual part from the large square into the two small squares by translations only. We have to use some rotations about 90° (clockwise). Figure 5 gives a dissection where this problem is fixed. But now the parts on the smallest square do no more fit in the global pattern of figure 3.

Fig. 5: Translation symmetry

Again the dissections in the two small squares have no symmetry.

4 Local symmetry

The dissection of figure 6 has symmetries in all the squares. But now the dissections in the small squares do not at all fit in the global pattern. We have only local symmetries in every square.

Every individual part of the largest square can be moved by translation only in the corresponding situation in one of the two smaller squares.

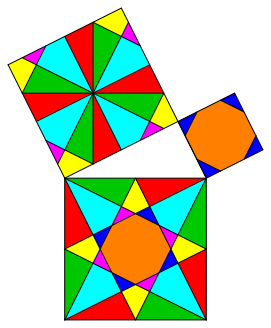
Fig. 6: Local symmetries

There are other solutions with the same properties like the solution of figure 6 (fig. 7, 8, and 9).

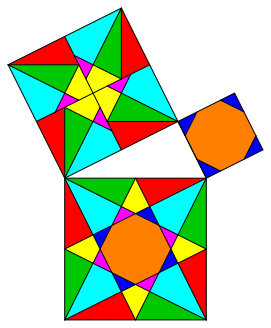
Fig. 7: Variation

Fig. 8: Variation

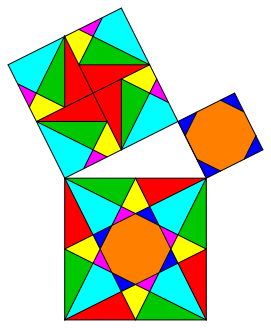
Fig. 9: Variation

The examples of figures 10 and 11 have less symmetry, but still local symmetry.

Fig. 10: Reduced local symmetry

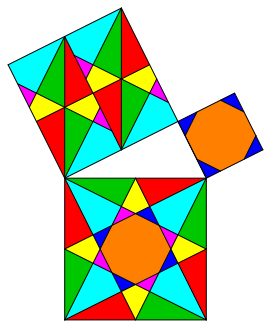
Fig. 11: Reduced symmetry