Hans Walser, [20130821]
Tribar Spirals
1 The Tribar
Figure 1 shows the usual tribar.

Fig. 1: The Tribar
The tribar is an impossible structure, it cannot be realized in the three dimensional world.
2 Spirals
Now we try to extend the tribar into spirals.
2.1 Archimedean Tribar Spiral
Figure 2 shows the Archimedean tribar spiral.

Fig. 2: Archimedean Tribar Spiral
We have a real end in the center. All branches of the spiral have he same width. This figure shows a not an impossible structure.
We explain this in a smaller version (Fig. 3). (The indicated numbers give the total lengths of the edges, if the structure is built from unit cubes. The edge lengths are multiples of three. )

Fig. 3: Smaller Version
In Figure 4 we have another view of the same structure. Clearly we see that this is possible in the three dimensional world.
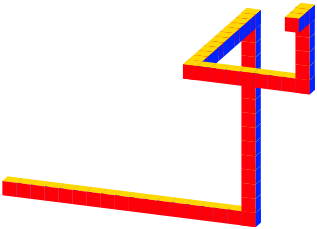
Fig. 4: Other View
2.2 Logarithmic Tribar Spiral
In Figure 5 we have a logarithmic tribar spiral. We have a point at infinity in the center.

Fig. 5: Logarithmic Tribar Spiral
Can you imagine that this Spiral is constructible in the three dimensional world?
Figure 6 gives another version. It goes down and down.
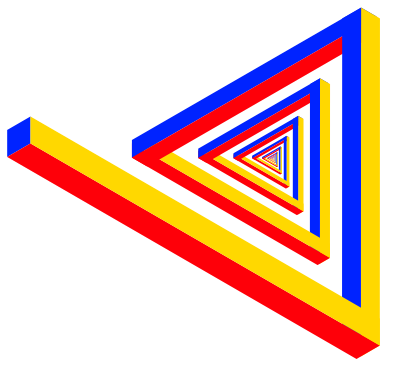
Fig. 6: Another Logarithmic Tribar Spiral
But the perspective in Figure 6 is not correct.
Figure 7 gives a better, but still not correct version.
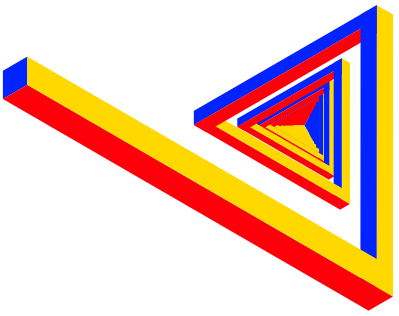
Fig. 7: Staircase to Hell